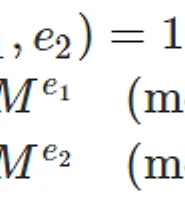Notice
Recent Posts
Recent Comments
Link
| 일 | 월 | 화 | 수 | 목 | 금 | 토 |
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | ||||
| 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| 11 | 12 | 13 | 14 | 15 | 16 | 17 |
| 18 | 19 | 20 | 21 | 22 | 23 | 24 |
| 25 | 26 | 27 | 28 | 29 | 30 | 31 |
Tags
- dreamhack
- bandit
- 암호학
- 드림핵
- spoofing
- picoCTF
- Montgomery Reduction
- Cube Root Attack
- weak key
- XSS
- rao
- RSA
- return address overflow
- OverTheWire Bandit Level 1 → Level 2
- 시스템해킹
- Franklin-Reiter Related Message Attack
- dns
- redirect
- Bandit Level 1 → Level 2
- AES
- CSRF
- arp
- 웹해킹
- Crypto
- shellcode
- RSA Common Modulas Attack
- pycrpytodome
- cryptography
- overthewire
- Hastad
Archives
- Today
- Total
암호(수학) 등.. 공부한 거 잊을거 같아서 만든 블로그
[Cryptography] Hastad's Broadcast Attack 본문
Hastad's Broadcast Attack
동일한 평문 M을 동일한 공개지수 e 를 사용하여 서로 다른 N값을 이용하여 암호화 한 값 C 알고 있을 때, 평문을 알아낼 수 있는 공격이다. (C, N 쌍이 e 크기 이상 만큼 필요하다.)
식의 크기를 줄이기 위해 공개 지수인 e의 값을 3이라 생각하고 하겠다.
아래의 C1, C2, C3, N1, N2, N3 를 알고 있다고 하자.
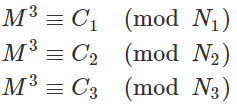
그러면 중국인의 나머지 정리 (CRT) 를 이용하여 M^3 mod N1 * N2 * N3 (M3 < N1 * N2 * N3) 의 값을 알아 낼 수 있다.
중국인의 나머지 정리를 이용하여 M^3를 구해보자.

위와 같이 M^3 mod N1 * N2 * N3 를 구할 수 있으며 M^3 < N1 * N2 * N3 이므로 이는 M^3 값이다.
M^3 < N1 * N2 * N3 인 이유
더보기
M^3 < N1 * N2 * N3 인 이유는 N1, N2, N3 가 2048비트라 하면, M은 최대 2047 비트 만큼의 크기를 가질 수 있다.
M^3 은 최대 2047 * 3 = 6141 비트 크기를 가질 수 있으며 N1*N2*N3 = 2048*3 = 6144 비트를 가진다.
따라서 M^3 < N1*N2*N3
이제 이 M^3 를 sagemath 의 nth_root 나 gmpy2 의 iroot 메서드를 이용해서 세제곱근을 구하여 M값을 알아낼 수 있다.
아래의 파이썬 코드는 Hastad's Broadcast Attack 의 예시 코드이다.
poc.py
# poc.py
from sage.all import *
from Crypto.Util.number import getPrime
from Crypto.Util.number import bytes_to_long, long_to_bytes
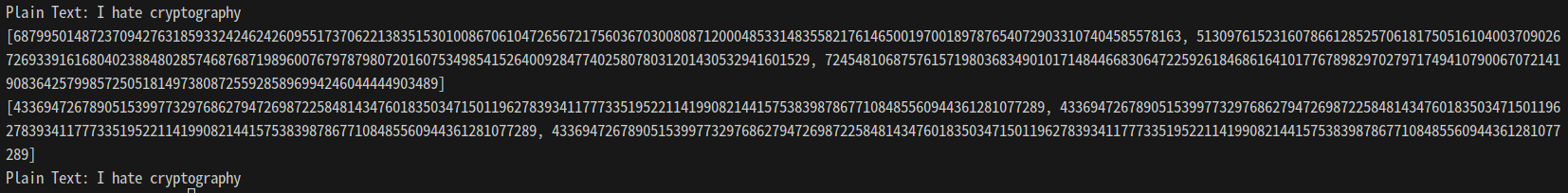
m = b"I hate cryptography"
print(f"Plain Text: {m.decode()}")
m = long_to_bytes(bytes_to_long(m))
# Key generation
N = [0, 0, 0]
for i in range(3):
p = getPrime(256)
q = getPrime(256)
N[i] = p * q
e = 3
# Encryption
c = [0, 0, 0]
for i in range(3):
c[i] = pow(bytes_to_long(m), e, N[i])
print(N)
print(c)
# Hastad's Broadcast Attack
M = N[0] * N[1] * N[2]
n1 = N[1] * N[2]
n2 = N[0] * N[2]
n3 = N[0] * N[1]
s1 = inverse_mod(n1, N[0])
s2 = inverse_mod(n2, N[1])
s3 = inverse_mod(n3, N[2])
decrypted = ((n1 * c[0] * s1) + (n2 * c[1] * s2) + (n3 * c[2] * s3)) % M
decrypted = decrypted.nth_root(3)
print("Plain Text:", long_to_bytes(decrypted).decode())
실행 결과
'Cryptography' 카테고리의 다른 글
| [Cryptography] Weak Key (0) | 2024.03.16 |
|---|---|
| [Cryptography] Franklin-Reiter Related Message Attack (0) | 2024.03.09 |
| [Cryptography] RSA Cube Root Attack (0) | 2024.02.03 |
| [Cryptography] RSA Common Modulas Attack (0) | 2024.01.27 |
| [Cryptograhpy] AES-ECB (Electronic Code Book) 운영 모드 (0) | 2023.07.07 |